| Title |
Anatomy of plants, with an idea of a philosophical history of plants, and several other lectures, read before the Royal Society. |
| Call Number |
QK41 .G82 |
| Date |
1692 |
| Description |
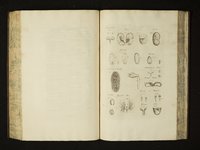
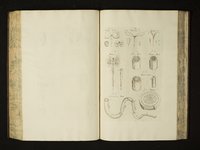
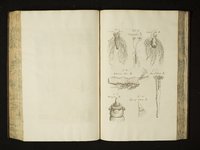
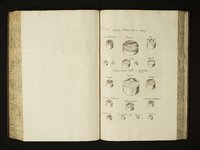
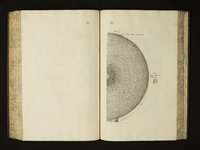
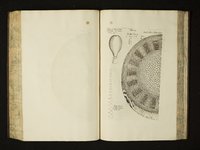
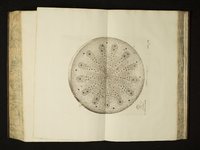
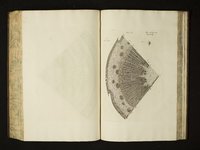
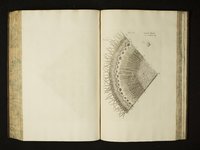
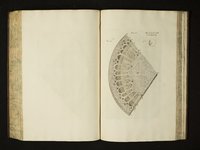
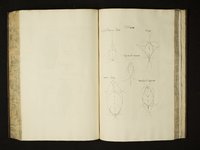
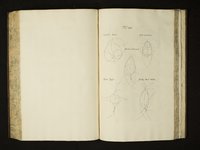
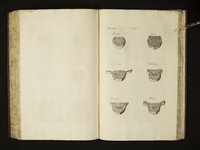
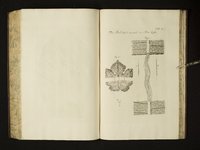
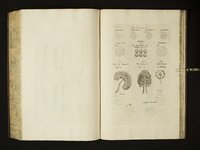
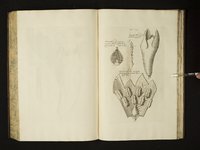
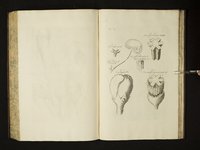
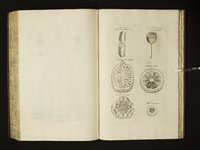
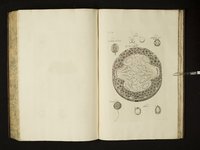
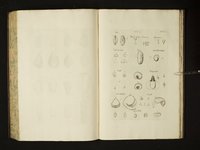
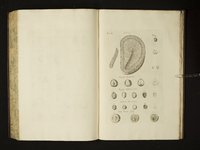
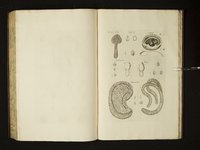
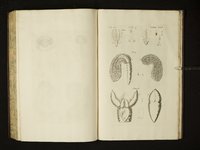
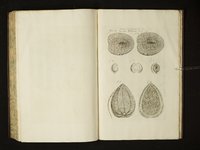
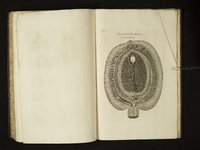
Originally printed in London by W. Rawlins, the work was largely a collection of previous publications. It was divided into four books, Anatomy of Vegetables begun, Anatomy of Roots, Anatomy of Trunks and Anatomy of Leaves, Flowers, Fruits and Seeds, and was illustrated with eighty-two plates, while appended to it were seven papers mostly of a chemical character. The Anatomy is especially notable for its descriptions of plant structure. He described nearly all the key differences of morphology of stem and root, showed that the flowers of the Asteraceae are built of multiple units, and correctly hypothesized that stamens are male organs. Anatomy of Plants also contains the first known microscopic description of pollen. |
| Creator |
Grew, Nehemiah, 1641-1712 |
| Subject |
Botany--Pre-Linnean works |
| Type |
Text |
| Format |
application/pdf |
| Language |
eng |
| Collection Name |
Rare Books Collection |
| Holding Institution |
Rare Books Division, Special Collections, J. Willard Marriott Library, University of Utah |
| Rights |
 |
| Scanning Technician |
Easton Madsen |
| ARK |
ark:/87278/s6jm81p3 |
| Setname |
uum_rbc |
| ID |
1609350 |
| Reference URL |
https://collections.lib.utah.edu/ark:/87278/s6jm81p3 |