| OCR Text |
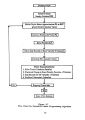
Show Minimize z = ^ £ QPm ( 2.17) t n 2) Minimize the operational costs of the well system Minimize Z = ] T £ CF ( QPm, L^) ( 2.18) t n where the cost is a function of withdrawal rate and average pump lift Ltn. Pumplift is a function of the Girinski potential. 3) Minimize the sum of the operation costs and the well installation costs and the well installation costs ( fixed costs). Minimize Z = ^ ^ C F ( QP^ L J + ] £ FC ( QPMn) ( 2.19) t n n Objective 1 utilizes total pumping as an economic measure, since the cost of operation is directly related to withdrawal rates. This objective allows a simple linear programming method to be used to obtain a solution. Objective 2 accounts for pumplift as well as withdrawal rate. The nonlinear nature of Objective 2 requires a solution method such as successive linear programming - Zhang et al ( 1985). Use of this objective function assumes that the time period for operating the well system be long enough for operating costs to be significant compared to installation costs. Objective 3 may be implemented when this assumption is invalid. A mixed- integer solution algorithm must normally be used in this case. For a nonlinear problem it is difficult to find successful mixed- integer algorithms for large problems. Constraints 1) The Girinski potential gmt, at points of interest m, should be at or below a desired level gmaxmt at the end of period t. gmt < gmax^ m « 1 .... m, t = 1 .... T ( 2.20) 2) The pumping rates, QPm » at w e^ n cannot exceed maximum pumping rates QPtn- QPm < QPMAXn n = 1 .... N, t = 1 .... T ( 2.21) 3) The response of the aquifer system to pumping at wells, n, is related to conditions at the beginning and end of period t, at points, m, where drawdown is of interest. « « - « m . t - l - 2 w Q P t n m - l . . . . M , J - l . . . . T ( 2.22) n where amnt are response matrix coefficients, for drawdowns in Girinski potential gmt at point m due to unit pumping at n. Constraints 1 and 3 may be combined to yield 18 |