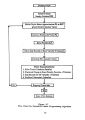
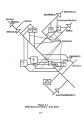
| OCR Text |
Show 5) The storage mass balance equation for each reservoir must be satisfied. RSti - RSt - 1 , i + ! ti ' X QRtil - X X biklt QPtkl " J > l - T ( 2.15) 1 k 1 where RSti is the storage in month t in reservoir i. Itj is the inflow ( including direct net precipitation input) into the i* h reservoir in month t. b ^ is the abstraction of water from reservoir i by unit pumping from the well ( l, k) 6) Limits on the storage capacity of a reservoir, RSj, must be met. RSMIR < RSa £ RSMAXi t - 1 ... T, i = 1... T ( 2.16) The resulting management model has constraints; L is the total number of water users/ agencies, M total number of points where drawdown is of concern, I the total number of reservoirs, J the total number of stream sections, N total number of pump locations and T total number of time periods. Decision variable include drawdown levels, pumping rates, reservoir storage levels, and stream and reservoir withdrawals. Response Matrix Development The response matrix coefficients, a ^ i t , in constraint 3 represent the aquifer response on drawdown at point m due to unit pumping at location kl. They are obtained by a simulation of the groundwater system using only one active well point at a time. The response matrix method is chosen in this formulation over the embedding method. The embedding method provides information on aquifer response at each node of the grid used in the finite difference or element discretization of the governing flow equations. This level of detail is generally not required for management of a groundwater system. Constraint of aquifer conditions at specific locations and time periods is a more common requirement. The response matrix method includes constraints for only these points. The resulting model can be run more economically, though initial costs, incurred from the simulations required to develop the response matrix, may be higher. The response matrix is an efficient way to represent aquifer response, and larger transient systems may be modeled using this method. The embedding approach can efficiently handle small steady problems, and those problems where constraints are called for at a large number of locations ( Gorelick, 1983). 2.2.2 Model for Dewatering Management of a dewatering site involves setting maximum levels of hydraulic head, then using an optimization model to determine pump locations, and pumping rates to maintain the desired drawdown. Planning periods must be considered to account for site expansion, eg. cases where an open pit mine is under development. An optimization model for least cost design of a dewatering scheme is presented. The objective of the optimization model is to minimize the cost of operating the well system. One of three objectives can be utilized to achieve this result. 1) Minimize the amount of pumping 17 |