| OCR Text |
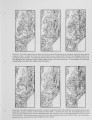
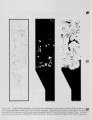
Show many of the performance limitations noted above as possible. b) identify specific recommendations based on these insights for possible modifications to the gas reburning system by which the reburning effectiveness could be improved, and c) obtain quantitative indications of the relative improvement in gas reburning effectiveness that can be expected from each of these recommendations, to allow rational decisions regarding possible implementation of various of these recommendations to be made. 3.1 LIM simulation geometry and initial conditions Though an extension of the LIM simulation code to fully three-dimensional problems is currently underway, at present the simulations are restricted to two-dimensional geometries, including planar and axisymmetric as well as swirling flows. However as indicated in §2, the burner blocks and SOFA system at Elrama Unit 2, as well as the wall injectors in the natural gas reburning system, can be reasonably modeled as a planar geometry. The LIM model geometry for the Elrama Unit 2 furnace is shown in Figs. 5a,b. All surfaces with the exception of inflows and outflows are represented by zero flow-through boundary conditions. Viscous effects at the walls are incorporated by vortex sheet shedding from all corners in the furnace interior. The coal and secondary air inflows through the burner are modeled as having the rearmost row of coal nozzles blocked on all burner blocks, as suggested by Fig. 2. The inflow rate through this section matches the actual coal and primary/secondary air inflows to the boiler. Detailed coal volatilization processes are not modeled; the coal inflows represent coal gas. The separated overfire air (SOFA) inflow is located as shown in Fig. 5b. Since primary interest is in the mixing and combustion properties within the furnace interior and with the injected natural gas from the reburn system, the convective pass is modeled as a simple duct. A uniform mass outflow matching the inflow rate is specified at the exit of the convective pass. Unlike time-averaged models, which only require boundary conditions. LIM simulations are time-dependent and thus begin from a set of initial conditions. The initial conditions correspond to the onset of fuel and air inflows into the initially quiescent furnace. For the flow field these are specified via vorticity surfaces that shed from all inflow edges, as well as from all corners in the furnace interior, and for the scalar field via scalar gradient surfaces that shed from the coal nozzles, as shown in Figs. 5a,b. These vorticity and scalar gradient surfaces, on which the LIM equations are solved, are completely free to move with the time-varying flow that results in the furnace interior, and thus are rapidly deformed by the underlying flow field, as is evident in Figs. 6a,b. As these surfaces deform with the flow field, at each time step the local deformation (strain) rate at each of the typically 20.000 or more points that define the surface enters the LIM equations for the local strain-diffusion balance to determine the evolution of the local moments at each of these points. The resulting flow and mixing fields can be coupled to a detailed reburn chemistry library that allows full GRI Mech 2.11 kinetics to be incorporated into the simulations. The resulting kinetic scheme uses 276 reactions involving 58 species. |